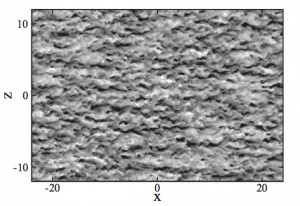
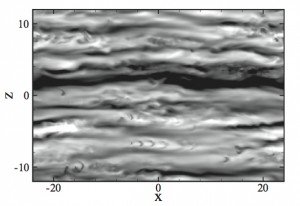
Unlike simple Newtonian fluids, complex viscoelastic fluids have a microstructure which endows them with macroscopic properties that defy our intuition. While a Newtonian fluid is depressed when stirred, a viscoelastic fluid climbs the rod; While a Newtonian fluid splashes against the surface, a viscoelastic jet bounces. In large-scale flows dominated by inertia, viscoelasticity is an effective strategy for reducing turbulent skin friction. The two top-views below contrast turbulence in channel flow of (left) Newtonian and (right) polymeric fluids.  The contours show the streamwise velocity perturbations.  Small-scale structures which are evident in the Newtonian flow are largely absent in the polymeric counterpart.

 

The dynamics of viscoelastic fluids have long remained mysterious. In this project we seek to further our understanding of their dynamics through a combination of fully non-linear direct numerical simulations and theoretical analyses.  By analyzing the simulation data, we are able to extract the relevant flow structures and isolate and explain the underlying mechanics using our analysis of the relevant canonical configurations.  For example, the videos below show the evolution of a weak spanwise vortex in homogeneous shear flow. The contours are the perturbation vorticity, and lines are the perturbation streamfunction. In the inviscid Newtonian fluid (left) the vorticity perturbations are advected by the base shear. In the weakly elastic fluid (centre) the vortex appears to split into a pair of new vortices. Furthermore, as the vortex is tilted forward under the action of the shear, the vorticity amplifies. This behaviour is impossible in a Newtonian fluid. Finally, in the strongly elastic fluid (right) the vortex splitting dominates, and the new vortices travel along the streamwise direction. This is a manifestation of elastic wave propagation along the tensioned mean-flow streamlines.